| Home |
| Blog |
| The Geek |
| Books |
| Movies |
| Video Games |
| Board Games |
| Music |
| Philip Reed |
| Older Posts > |

 | 28th July, 2018 |  | ||||||||
 |  |  | ||||||||
 | Some images from a Minecraft server I used to play on:
|  | ||||||||
 |  |  | ||||||||

 | 28th May, 2018 |  | ||
 |  |  | ||
 | I've seen a couple of things like this online, but I thought I'd create one of my own: a questionnaire tabletop RPG groups and GM's can use to get an idea as to what everyone is looking for out of the game. It's very easy for groups to get together and, after a few sessions, realise everyone's playing styles or preferences don't gel, or don't fit the setting the GM has come up with. Gathering info from the players ahead of time can help guide which RPG system to play, the settings of the world and the campaign they want to be involved in, as well as pertinent out of game information. With that in mind, here's my thoughts of a questionnaire that could help: Are You Interested In Being Involved (As Player Or GM) In A Traditional / "Tabletop" Roleplaying Game? (GM = Game Master, aka DM or Dungeon Master, NPC = Non-Player Character, an in-game character controlled by the GM) In The Game: The Genre Or Setting Of The Game: SETTING ["1" (really not interested) to "5" (very interested)]:
CREATURES ["1" (really not interested) to "5" (very interested)]:
ABILITIES ["1" (really not interested) to "5" (very interested)]:
WEAPONS ["1" (really not interested) to "5" (very interested)]:
TRAVEL ["1" (really not interested) to "5" (very interested)]:
Outside The Game: The Mechanics Or Meta Of The Game: CAMPAIGN ["1" (strongly disagree) to "5" (strongly agree)]:
CHARACTERS ["1" (strongly disagree) to "5" (strongly agree)]:
GROUP ["1" (strongly disagree) to "5" (strongly agree)]:
RULESET ["1" (strongly disagree) to "5" (strongly agree)]:
ME ["1" (strongly disagree) to "5" (strongly agree)]:
Finally:
|  | ||
 |  |  | ||

 | 7th April, 2013 |  | ||
 |  |  | ||
 | So, recently I just finished reading The Picture of Dorian Gray the other week. My conclusion: I don't think I would have liked Oscar Wilde as a person much. I've never read the book before, and the majority of my previous knowledge about Oscar Wilde is based on the trials that focused on his personal life. A victim of the era he was born into, a can sympathise with him from that angle. However, as an author, this book has not endeared me to his writing style. Okay, it's clearly provocative, again riling against the tight laced practices of the Victorian age. However, for a book that is clearly striving to highlight and promote the concepts of decadence, flamboyance, and art over practicality, I found the book itself to border on tedium in too many places. There's a whole chapter which is just clearly Wilde boasting of his own literary knowledge, which is boring in the extreme. There seems no real redemption for any of the flawed characters (which gives no reason for me to care as to whether they come to a good or bad end), and they all deride the working class and practical professions of the time, without the narrative giving any indication that the reader should believe otherwise themselves. There's a period where almost two decades pass and we're only given the flimsiest of suggestions that he's done "bad things" in the intervening years, and the book ends too suddenly after Dorian's death. All in all, I feel like this is a self indulgent book by the author where the reader comes away feeling as if Wilde wishes he could have been more Dorian-like himself. It's worth reading because of its classic status and to maybe get an idea of the clash of certain Victorian cultures, but I know I won't be reading this again, and I'm less sympathetic towards its author than I was before picking it up. |  | ||
 |  |  | ||

 | 30th March, 2013 |  | |||
 |  |  | |||
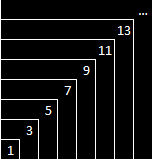
 | Is there anything remarkable about the fact that 32 + 42 = 52? A couple of random mathematical conversations occurred at work the other week, during one of which the above equation was mentioned (it's a commonly known integer solution for a right angle triangle). It was noted that some people find it too coincidental that things like the above are true. Personally, I'm of the reverse opinion. There's a million other equations we could test for small numbers that wouldn't turn out to be true, it's just this one is. It might seem interesting, but is there really anything unique about it...? Let's have a meandering chat about some mathematical concepts: There's An Infinite Number Of Numbers This is a pretty trivial statement that most people come across early in their school days. The proof? It's simply that if you think there's a "biggest number" we can always add 1 to it to make an even bigger one. For ever. Not much to say here, but let's throw around some basic stuff first. The Sum Of The First N Odd Numbers Is A Square This is another one that's relatively simple to demonstrate:
1 = 12 = 1 Basically, start from 1 and add the next odd number, and the next, and the next, and... Do this as many times as you like and you will always end up with a square number. Further, for any odd number there's some square number which if you add that odd number to it will also result in a square (e.g. for odd number 13, 72 = 62 + 13). Combine The Two So, what can get from the two topics above? Well, from the first point we know there's obviously an infinite amount of odd numbers. Let's call one of those numbers X. X2 is also an odd number. And from our second point, we know there's always some square number Y2 such that the (Y+1)2 = Y2 + X2. With a bit of magic maths, we can get a bit further than this too. I'll leave it as an exercise for the reader, but with some number crunching we can relatively easily determine that for a given X in the above equation, Y = (X2 - 1) / 2. Let's start calculating some of these: 52 = 42 + 32 So, basically, our original formula is just one of an infinite number of "right angled triangle" solutions, all following a regular pattern where the two longest sides differ by just one unit. In Summary You might still feel that 3/4/5 is still worthy of consideration as being a special and unique case though. After all, no other solutions form a sequence of three numbers, right? And it's the first solution for the equation? Well, maybe... If it weren't for that fact that 12 = 02 + (-1)2. Recommended further reading: Fermat's Last Theorem |  | |||
 |  |  | |||

| Older Posts > |